Vito Scarola
Professor of Physics

Work in our group spans several subfields of theoretical quantum physics with the aim of fostering quantum state engineering in the laboratory. The pristine environments we study typically allow for close connection with experiment in, e.g., two dimensional materials as well as atomic, molecular, and optical (AMO) systems. Recent research directions include algorithms for quantum simulation, modelling of quantum computing hardware, quantum analogue simulation, and topological states of matter.
Algorithms for Quantum Simulation:
Exact results on otherwise intractable quantum problems have been long sought in a diverse array of fields including: quantum chemistry, materials science, high energy physics, and solid-state physics. Unfortunately, the complexity of quantum many-body problems has left long-standing open problems. Quantum algorithms can be used to speed up calculations on quantum many-body problems. Work in our group constructs and examines quantum algorithms designed to solve important quantum many-body problems.
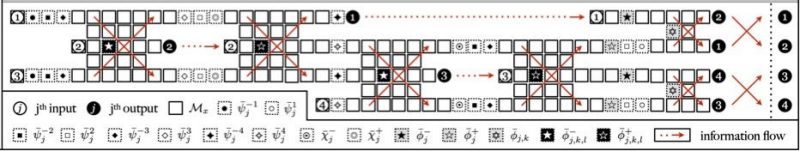
Phys. Rev. Research 4, L032013 (2022).
Quantum Computing Hardware:
Semiconductor Devices-Semiconductor devices offered early and promising platforms for quantum state engineering because they leverage expertise in the semiconductor industry. This led to quantum computing proposals that blossomed into large international efforts. For example, electron spins in semiconductor quantum dots can yield tunable qubits. Our work examines the role of electron-electron interactions in harnessing and potentially even harming quantum information stored in quantum dot qubits.
Phys. Rev. A 71, 032340 (2005)
Phys. Rev. Lett. 93, 120503 (2004)
Phys. Rev. Lett. 91, 167903 (2003)
Atomic and Molecular Qubits-Progress in atomic clock technology established ions and neutral atoms as competitive qubit technologies. Long lifetimes, high precision, and optical addressing set up these platforms as ideal candidates for quantum computing. Our work models atomic and molecular systems for use in quantum information processing. For example, we proposed a route to create high fidelity quantum registers from neutral atom Mott insulators. More recently, the community has begun to explore the possibility of using ultra-cold molecules as qubits. Work in our group is currently modelling molecules trapped in optical tweezers to support ongoing laboratory efforts to use these systems to build large quantum states.
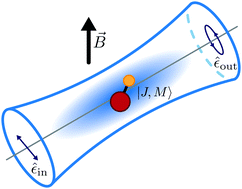
Quantum Analogue Simulation
Quantum analogue simulation complements digital quantum computation by using continuous degrees of freedom to reconstruct, and in some cases, replicate otherwise intractable models and phenomena. Running the simulator and extracting an observable is tantamount to solving the problem being explored by, for example, mapping out phase diagrams. One way to build a simulator of an important but intractable model is to ensure that a physical system is very well parameterized by the model of interest. AMO systems offer excellent platforms for quantum analogue simulation because of precise control and tunability of system parameters. Our work has shown how the precise control over parameters in AMO systems allows simulation of large classes of models of interest to many areas of physics.

See a highlight of our research in: Nature News and Views .
Topological States of Matter
Topologically ordered many-body states of matter carry unique properties such as fractionalized excitations and chiral edge currents, properties that are intimately connected to the topology of the underlying state. Examples of topologically ordered many-body states include fractional quantum Hall states or topological superconductors.
Some topological states can serve as platforms for a robust route to quantum computing. In topologically ordered systems, excitations are anyons which can be thought of as qubits. Braiding anyons in time and space executes quantum gates that do not necessarily need conventional error correction overhead. Topological quantum computing research is therefore a rich topic of fundamental scientific importance with concomitant applications to quantum information science.
Work in our group on this topic centers on establishing topologically ordered ground states as viable in the laboratory. We have focused on two platforms in particular: the fractional quantum Hall regime and in atomic systems.

See highlights of our research in Nature News and Views and in Science
Selected Works
Quantum Phases of the Extended Bose-Hubbard Hamiltonian:
The Possibility of a Supersolid State of Cold Atoms in Optical Lattices,
V. W. Scarola and S. Das Sarma,
Phys. Rev. Lett. 95 , 033003 (2005).
Dispersion of the Excitations of Fractional Quantum Hall States,
I. V. Kukushkin, J. H. Smet, V. W. Scarola, V. Umansky, and K. von Klitzing,
Science 324, 1044 (2009).
Cooper Instability of Composite Fermions,
V. W. Scarola, K. Park, and J.K. Jain,
Nature 406, 863 (2000)
Research Funding





